This project seeks to construct models for the study of upper ocean phenomena with two characteristics: 1) to be capable of incorporating thermodynamic processes while maintaining the 2-d structure of the rotating shallow-water equations, a paradigm of ocean dynamics on scales longer than a few hours; and 2) to preserve the geometric (generalized Hamiltonian/Euler–Poincare) structure of the exact 3-d models from which they derive. Characteristic 1) promises fundamental understanding of ocean processes difficult---if not impossible—--to be attained using general circulation models. Characteristic 2) facilitates the discovery of new dynamics consistent with observations through the application of recent advances in transparent machine learning.
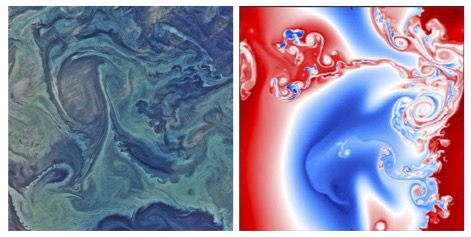Figure. (left panel) Ocean color image acquired by VIIRS (Visible Infrared Imaging Radiometer Suite) on 1-1-2015 west of the Drake Passage in the Southern Ocean, revealing vortices with diameters ranging from a couple of km to a couple of hundred km. Image credit: NASA Ocean Color Web (https://oceancolor.gsfc.nasa.gov/gallery/447/). (right panel) Kelvin–Helmholtz-like vortices manifesting along a density gradient in a direct numerical simulation of the IL0QG, within a doubly periodic domain with a side length corresponding to the baroclinic deformation radius. From [Beron-Vera, 2021c].
The work builds on two existing thermal shallow-water theories. One theory enjoys the required geometric characteristic, and is capable of producing circulations akin to submesoscale circulatory motions observed using satellites, even at low frequency. This illustrated in the figure below. However, this theory falls short at representing important mixed-layer phenomena such as restratification induced by baroclinic instability. The second theory represents an important improvement over the first theory in that it is capable of simulating mixed-layer restratification, yet it does not possess the needed geometric structure. To derive a theory that it is capable of describing the tendency of buoyancy gradients to slump from the horizontal to the vertical in a geometrically consistent manner, one can start from the Hamilton’s principle for the Euler-Boussinesq equations for stratified fluid, and make appropriate approximations (e.g., truncations in the vertical structure of the dynamical fields) in the associated action functional so that the underlying semidirect-product Lie algebra structure is preserved.
References
- Beron-Vera, F. J. and E. Luseink (2025). Dual Euler–Poincaré/Lie–Poisson formulation of subinertial stratified thermal ocean flow with identification of Casimirs as Noether quantities. J. Math. Phys., submitted.
- Beron-Vera, F. J. and M. J. Olascoaga (2025). Properties and baroclinic instability of stratified thermal upper-ocean flow. Rev. Mex. Fís., submitted.
- Beron-Vera, F. J. (2024). On a priori bounding the growth of thermal instability amplitudes. Phys. Fluids 36, 041702.
- Beron-Vera, F. J. (2021). Extended rotating shallow-water theories with thermodynamics and geometry. Phys. Fluids 33, 106605.
- Beron-Vera, F. J. (2021). Multilayer shallow-water model with stratification and shear. Rev. Mex. Fís. 67, 351–364.
- Beron-Vera, F. J. (2021). Nonlinear saturation of thermal instabilities. Phys. Fluids 33, 036608.
